Atomic Structure Guided Reading Answers the Discovery of Atomic Structure

2.1: A History of Atomic Theory

1. Final updated

2. Save every bit PDF

Page ID

98684

Skills to Develop

Past the cease of this department, yous will be able to:

• State the postulates of Dalton's diminutive theory
• Utilize postulates of Dalton'due south atomic theory to explain the laws of definite and multiple proportions
• Outline milestones in the development of modern atomic theory
• Summarize and interpret the results of the experiments of Thomson, Millikan, and Rutherford

A Video Introduction to Atomic Theory through the Nineteenth Century From Crash Course Chemistry

Video \(\PageIndex{1}\): Lavoisier's discovery of The Law of Conservation of Affair led to the Laws of Definite and Multiple Proportions and eventually Dalton's Diminutive Theory.

Diminutive Theory through the Nineteenth Century

The earliest recorded discussion of the basic structure of thing comes from ancient Greek philosophers, the scientists of their day. In the fifth century BC, Leucippus and Democritus argued that all thing was equanimous of small, finite particles that they called atomos, a term derived from the Greek word for "indivisible." They idea of atoms as moving particles that differed in shape and size, and which could join together. Afterward, Aristotle and others came to the conclusion that matter consisted of various combinations of the four "elements"—burn, earth, air, and water—and could be infinitely divided. Interestingly, these philosophers thought about atoms and "elements" as philosophical concepts, but obviously never considered performing experiments to examination their ideas.

The Aristotelian view of the composition of matter held sway for over 2 k years, until English schoolteacher John Dalton helped to revolutionize chemical science with his hypothesis that the behavior of matter could be explained using an diminutive theory. Commencement published in 1807, many of Dalton's hypotheses about the microscopic features of matter are still valid in modern atomic theory. Hither are the postulates of Dalton's diminutive theory.

1. Matter is equanimous of exceedingly small particles called atoms. An atom is the smallest unit of an element that can participate in a chemical change.
2. An element consists of merely one blazon of cantlet, which has a mass that is characteristic of the element and is the aforementioned for all atoms of that element (Figure \(\PageIndex{1}\)). A macroscopic sample of an element contains an incredibly big number of atoms, all of which accept identical chemic properties.
3. Atoms of one element differ in properties from atoms of all other elements.
4. A chemical compound consists of atoms of two or more elements combined in a small, whole-number ratio. In a given compound, the numbers of atoms of each of its elements are always present in the same ratio (Effigy \(\PageIndex{two}\)).
5. Atoms are neither created nor destroyed during a chemic change, only are instead rearranged to yield substances that are different from those present before the change (Effigy \(\PageIndex{iii}\)).

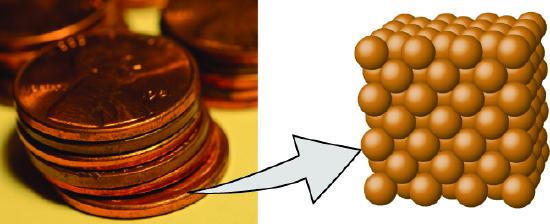
Figure \(\PageIndex{one}\): A pre-1982 copper penny (left) contains approximately 3 \(\times\) 10²² copper atoms (several dozen are represented as dark-brown spheres at the right), each of which has the same chemical properties. (credit: modification of work by "slgckgc"/Flickr)

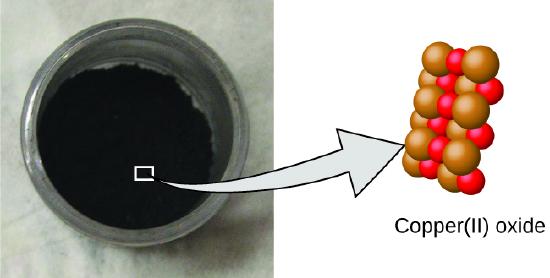
Figure \(\PageIndex{2}\): Copper(Ii) oxide, a powdery, black compound, results from the combination of ii types of atoms—copper (brown spheres) and oxygen (red spheres)—in a 1:1 ratio. (credit: modification of piece of work past "Chemicalinterest"/Wikimedia Commons)

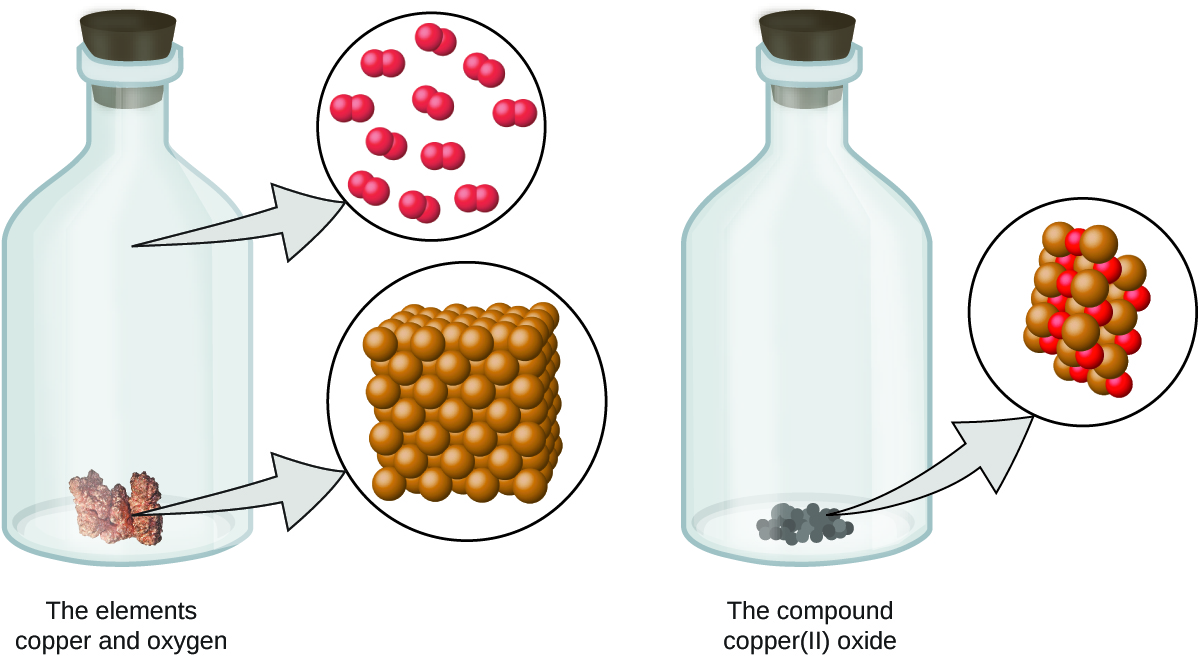
Figure \(\PageIndex{3}\): When the elements copper (a shiny, red-brownish solid, shown hither as brown spheres) and oxygen (a clear and colorless gas, shown hither as red spheres) react, their atoms rearrange to form a compound containing copper and oxygen (a powdery, black solid). (credit copper: modification of work by http://images-of-elements.com/copper.php).

Dalton'southward atomic theory provides a microscopic explanation of the many macroscopic properties of thing that you've learned nigh. For example, if an element such every bit copper consists of just ane kind of atom, and so it cannot be cleaved down into simpler substances, that is, into substances composed of fewer types of atoms. And if atoms are neither created nor destroyed during a chemical alter, and so the total mass of thing nowadays when matter changes from 1 type to another volition remain constant (the law of conservation of thing (or mass)).

Want to larn more than virtually the Police of Conservation of Mass?

Video \(\PageIndex{2}\): "We are made of star stuff" - Carl Sagan .

Case \(\PageIndex{1}\): Testing Dalton'southward Atomic Theory

In the post-obit drawing, the green spheres correspond atoms of a certain element. The imperial spheres represent atoms of another element. If the spheres touch, they are part of a single unit of a compound. Does the following chemical change represented by these symbols violate any of the ideas of Dalton's diminutive theory? If so, which one?

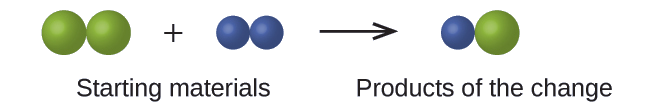
Solution

The starting materials consist of two light-green spheres and two purple spheres. The products consist of only one green sphere and one royal sphere. This violates Dalton's postulate that atoms are neither created nor destroyed during a chemical change, but are only redistributed. (In this instance, atoms appear to have been destroyed.)

Exercise \(\PageIndex{1}\)

In the following cartoon, the greenish spheres correspond atoms of a certain chemical element. The purple spheres stand for atoms of another element. If the spheres touch, they are part of a unmarried unit of measurement of a compound. Does the following chemical change represented by these symbols violate whatever of the ideas of Dalton's atomic theory? If so, which i

Answer

The starting materials consist of four greenish spheres and 2 imperial spheres. The products consist of four green spheres and 2 purple spheres. This does non violate whatsoever of Dalton's postulates: Atoms are neither created nor destroyed, but are redistributed in small, whole-number ratios.

Dalton knew of the experiments of French chemist Joseph Proust, who demonstrated that all samples of a pure compound contain the same elements in the same proportion past mass. This statement is known as the law of definite proportions or the law of constant limerick. The proposition that the numbers of atoms of the elements in a given compound always exist in the same ratio is consistent with these observations. For example, when different samples of isooctane (a component of gasoline and one of the standards used in the octane rating system) are analyzed, they are found to take a carbon-to-hydrogen mass ratio of 5.33:1, as shown in Tabular array \(\PageIndex{one}\).

Table \(\PageIndex{1}\): Constant Composition of Isooctane

Sample	Carbon	Hydrogen	Mass Ratio
A	xiv.82 g	2.78 k	\(\mathrm{\dfrac{14.82\: g\: carbon}{two.78\: thousand\: hydrogen}=\dfrac{5.33\: g\: carbon}{1.00\: one thousand\: hydrogen}}\)
B	22.33 thou	iv.19 one thousand	\(\mathrm{\dfrac{22.33\: g\: carbon}{4.19\: grand\: hydrogen}=\dfrac{five.33\: thousand\: carbon}{1.00\: thou\: hydrogen}}\)
C	19.40 one thousand	three.64 g	\(\mathrm{\dfrac{19.40\: k\: carbon}{3.63\: g\: hydrogen}=\dfrac{5.33\: g\: carbon}{ane.00\: g\: hydrogen}}\)

It is worth noting that although all samples of a detail chemical compound have the aforementioned mass ratio, the converse is not true in general. That is, samples that have the same mass ratio are non necessarily the same substance. For example, in that location are many compounds other than isooctane that also have a carbon-to-hydrogen mass ratio of v.33:1.00.

Dalton also used data from Proust, as well every bit results from his own experiments, to codify another interesting police force. The constabulary of multiple proportions states that when 2 elements react to course more than than one chemical compound, a fixed mass of i element will react with masses of the other element in a ratio of small, whole numbers. For example, copper and chlorine can form a greenish, crystalline solid with a mass ratio of 0.558 g chlorine to one grand copper, as well every bit a brownish crystalline solid with a mass ratio of i.116 chiliad chlorine to 1 grand copper. These ratios by themselves may not seem peculiarly interesting or informative; notwithstanding, if we take a ratio of these ratios, we obtain a useful and possibly surprising event: a small, whole-number ratio.

\[\mathrm{\dfrac{\dfrac{1.116\: one thousand\: Cl}{1\: m\: Cu}}{\dfrac{0.558\: thousand\: Cl}{1\: g\: Cu}}=\dfrac{ii}{one}}\]

This ii-to-1 ratio means that the chocolate-brown compound has twice the amount of chlorine per amount of copper as the light-green compound.

This can be explained by atomic theory if the copper-to-chlorine ratio in the brown compound is 1 copper cantlet to 2 chlorine atoms, and the ratio in the green compound is 1 copper atom to i chlorine cantlet. The ratio of chlorine atoms (and thus the ratio of their masses) is therefore two to 1 (Figure \(\PageIndex{4}\)).

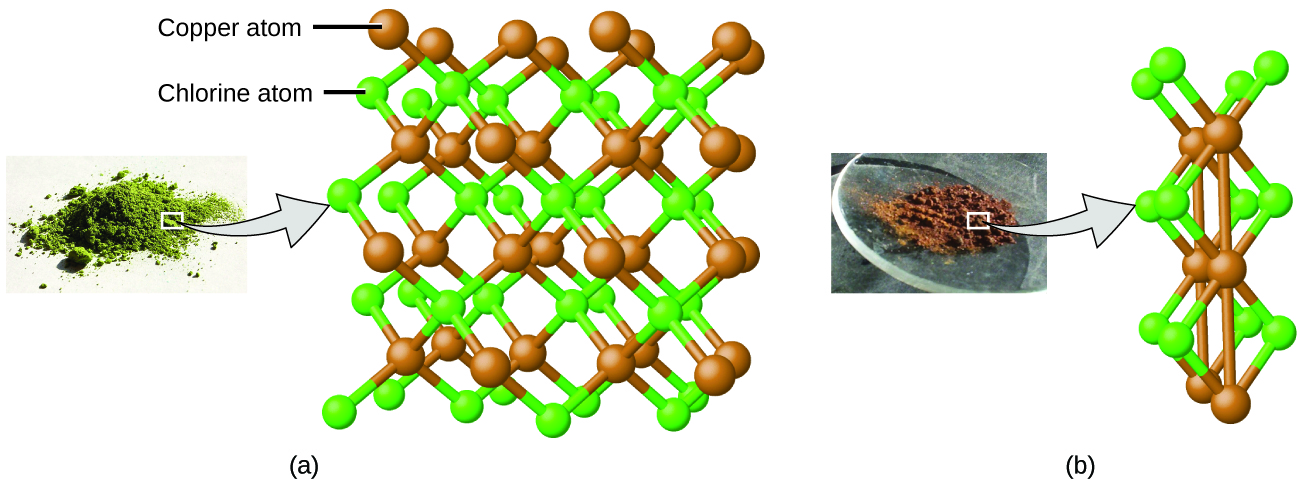
Figure \(\PageIndex{4}\): Compared to the copper chlorine compound in (a), where copper is represented by brown spheres and chlorine by light-green spheres, the copper chlorine compound in (b) has twice every bit many chlorine atoms per copper atom. (credit a: modification of work by "Benjah-bmm27"/Wikimedia Commons; credit b: modification of work past "Walkerma"/Wikimedia Eatables)

Example \(\PageIndex{2}\): Laws of Definite and Multiple Proportions

A sample of chemical compound A (a clear, colorless gas) is analyzed and establish to contain four.27 chiliad carbon and 5.69 chiliad oxygen. A sample of compound B (as well a clear, colorless gas) is analyzed and institute to contain 5.19 thousand carbon and 13.84 thousand oxygen. Are these data an instance of the law of definite proportions, the law of multiple proportions, or neither? What do these data tell you about substances A and B?

Solution

In chemical compound A, the mass ratio of carbon to oxygen is:

\[\mathrm{\dfrac{ane.33\: g\: O}{1\: g\: C}} \nonumber\]

In compound B, the mass ratio of carbon to oxygen is:

\[\mathrm{\dfrac{2.67\: g\: O}{1\: g\: C}} \nonumber\]

The ratio of these ratios is:

\[\mathrm{\dfrac{\dfrac{i.33\: g\: O}{one\: m\: C}}{\dfrac{2.67\: g\: O}{ane\: g\: C}}=\dfrac{one}{ii}} \nonumber\]

This supports the police force of multiple proportions. This means that A and B are different compounds, with A having ane-one-half as much carbon per amount of oxygen (or twice every bit much oxygen per amount of carbon) as B. A possible pair of compounds that would fit this human relationship would exist A = CO₂ and B = CO.

Do \(\PageIndex{2}\)

A sample of chemical compound X (a clear, colorless, combustible liquid with a noticeable odor) is analyzed and establish to incorporate fourteen.13 g carbon and 2.96 g hydrogen. A sample of compound Y (a clear, colorless, combustible liquid with a noticeable odor that is slightly different from X's smell) is analyzed and found to comprise 19.91 g carbon and three.34 g hydrogen. Are these data an example of the law of definite proportions, the law of multiple proportions, or neither? What do these data tell you nearly substances X and Y?

Answer

In compound X, the mass ratio of carbon to hydrogen is \(\mathrm{\dfrac{14.13\: m\: C}{2.96\: yard\: H}}\).

In compound Y, the mass ratio of carbon to oxygen is \(\mathrm{\dfrac{19.91\: g\: C}{3.34\: g\: H}}\).

The ratio of these ratios is

\[\mathrm{\dfrac{\dfrac{14.13\: g\: C}{two.96\: g\: H}}{\dfrac{19.91\: g\: C}{3.34\: m\: H}}=\dfrac{4.77\: g\: C/g\: H}{5.96\: g\: C/g\: H}=0.800=\dfrac{four}{v}}. \nonumber\]

This pocket-size, whole-number ratio supports the law of multiple proportions. This ways that X and Y are different compounds.

In the ii centuries since Dalton developed his ideas, scientists take made pregnant progress in furthering our understanding of atomic theory. Much of this came from the results of several seminal experiments that revealed the details of the internal structure of atoms. Here, we will discuss some of those key developments, with an emphasis on application of the scientific method, likewise equally understanding how the experimental show was analyzed. While the historical persons and dates behind these experiments can be quite interesting, it is most of import to understand the concepts resulting from their work.

Atomic Theory later on the Nineteenth Century

If matter were composed of atoms, what were atoms equanimous of? Were they the smallest particles, or was there something smaller? In the late 1800s, a number of scientists interested in questions like these investigated the electrical discharges that could be produced in low-pressure level gases, with the virtually significant discovery fabricated by English physicist J. J. Thomson using a cathode ray tube. This appliance consisted of a sealed glass tube from which almost all the air had been removed; the tube contained two metal electrodes. When high voltage was applied across the electrodes, a visible beam chosen a cathode ray appeared between them. This beam was deflected toward the positive charge and away from the negative accuse, and was produced in the aforementioned manner with identical backdrop when dissimilar metals were used for the electrodes. In similar experiments, the ray was simultaneously deflected by an practical magnetic field, and measurements of the extent of deflection and the magnetic field force allowed Thomson to calculate the accuse-to-mass ratio of the cathode ray particles. The results of these measurements indicated that these particles were much lighter than atoms (Figure \(\PageIndex{one}\)).

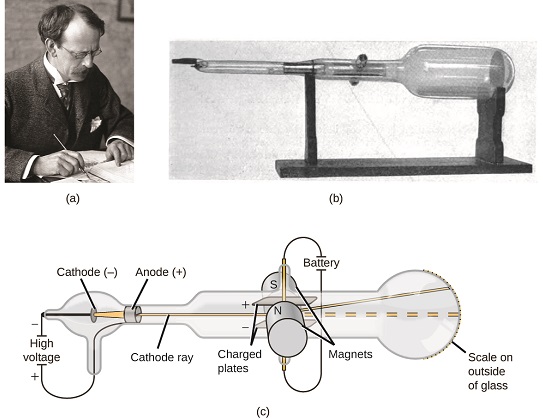
Figure \(\PageIndex{5}\): (a) J. J. Thomson produced a visible beam in a cathode ray tube. (b) This is an early cathode ray tube, invented in 1897 by Ferdinand Braun. (c) In the cathode ray, the beam (shown in xanthous) comes from the cathode and is accelerated past the anode toward a fluorescent scale at the terminate of the tube. Simultaneous deflections by applied electrical and magnetic fields permitted Thomson to summate the mass-to-charge ratio of the particles composing the cathode ray. (credit a: modification of work by Nobel Foundation; credit b: modification of work past Eugen Nesper; credit c: modification of work by "Kurzon"/Wikimedia Commons).

Based on his observations, here is what Thomson proposed and why: The particles are attracted by positive (+) charges and repelled by negative (−) charges, then they must be negatively charged (similar charges repel and unlike charges attract); they are less massive than atoms and indistinguishable, regardless of the source textile, so they must be fundamental, subatomic constituents of all atoms. Although controversial at the time, Thomson'southward thought was gradually accepted, and his cathode ray particle is what we now phone call an electron, a negatively charged, subatomic particle with a mass more than 1 chiliad-times less that of an cantlet. The term "electron" was coined in 1891 past Irish gaelic physicist George Stoney, from "electric ion."

In 1909, more information about the electron was uncovered by American physicist Robert A. Millikan via his "oil drib" experiments. Millikan created microscopic oil aerosol, which could be electrically charged by friction as they formed or by using X-rays. These droplets initially fell due to gravity, simply their downward progress could be slowed or even reversed by an electric field lower in the appliance. By adjusting the electric field force and making careful measurements and advisable calculations, Millikan was able to determine the charge on individual drops (Figure \(\PageIndex{2}\)).

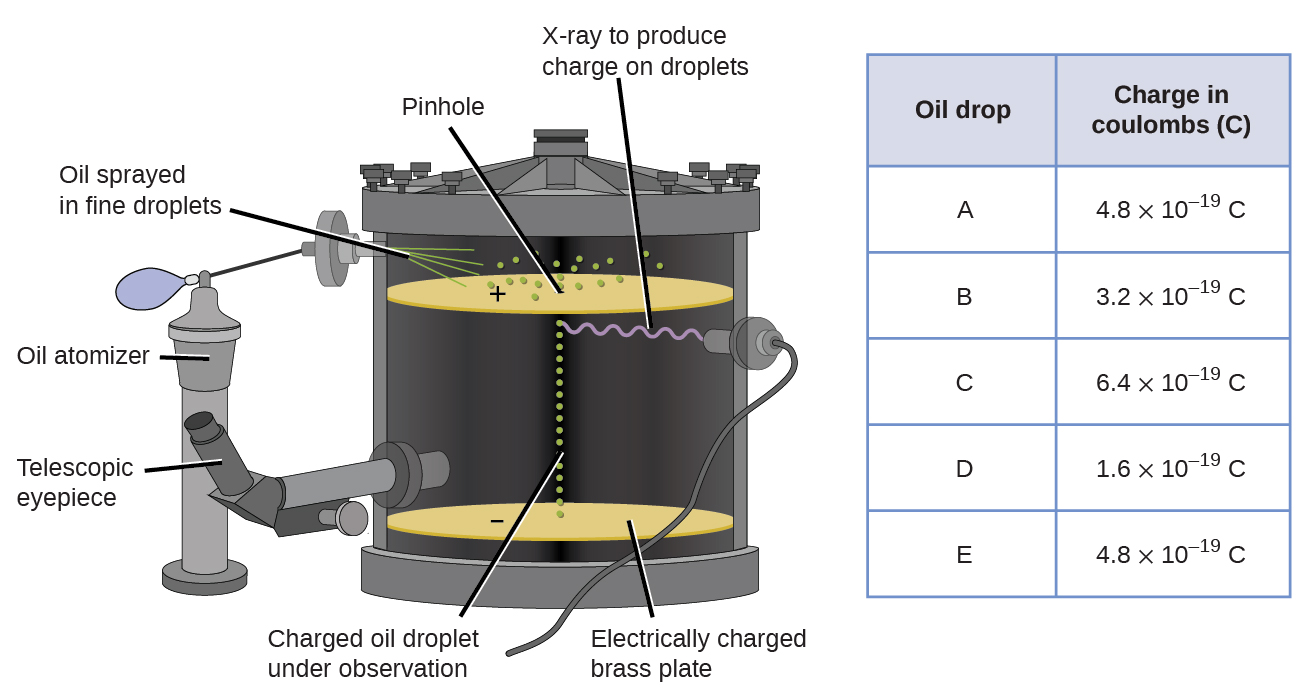
Effigy \(\PageIndex{6}\): Millikan'south experiment measured the charge of individual oil drops. The tabulated data are examples of a few possible values.

Looking at the charge information that Millikan gathered, you may have recognized that the charge of an oil droplet is always a multiple of a specific charge, i.vi \(\times\) ten^−xix C. Millikan ended that this value must therefore be a fundamental charge—the accuse of a single electron—with his measured charges due to an excess of one electron (1 times 1.half dozen \(\times\) ten^−xix C), ii electrons (2 times 1.half dozen \(\times\) ten⁻¹⁹ C), three electrons (iii times i.6 \(\times\) ten⁻¹⁹ C), and then on, on a given oil droplet. Since the charge of an electron was now known due to Millikan's research, and the accuse-to-mass ratio was already known due to Thomson'south research (1.759 \(\times\) 10^eleven C/kg), information technology but required a simple calculation to decide the mass of the electron also.

\[\mathrm{Mass\: of\: electron=i.602\times 10^{-19}\:\cancel{C}\times \dfrac{1\: kg}{one.759\times 10^{xi}\:\cancel{C}}=9.107\times x^{-31}\:kg} \tag{2.3.1}\]

Scientists had now established that the cantlet was not indivisible every bit Dalton had believed, and due to the work of Thomson, Millikan, and others, the charge and mass of the negative, subatomic particles—the electrons—were known. However, the positively charged part of an cantlet was non notwithstanding well understood. In 1904, Thomson proposed the "plum pudding" model of atoms, which described a positively charged mass with an equal amount of negative charge in the form of electrons embedded in it, since all atoms are electrically neutral. A competing model had been proposed in 1903 by Hantaro Nagaoka, who postulated a Saturn-similar atom, consisting of a positively charged sphere surrounded by a halo of electrons (Figure \(\PageIndex{3}\)).

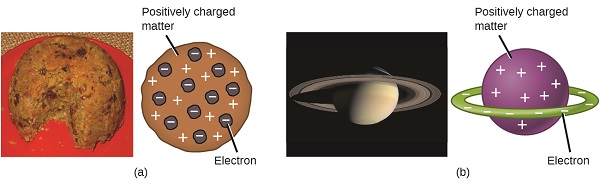
Effigy \(\PageIndex{7}\): (a) Thomson suggested that atoms resembled plum pudding, an English dessert consisting of moist cake with embedded raisins ("plums"). (b) Nagaoka proposed that atoms resembled the planet Saturn, with a band of electrons surrounding a positive "planet." (credit a: modification of piece of work by "Man vyi"/Wikimedia Eatables; credit b: modification of work by "NASA"/Wikimedia Commons).

The next major evolution in understanding the atom came from Ernest Rutherford, a physicist from New Zealand who largely spent his scientific career in Canada and England. He performed a series of experiments using a beam of loftier-speed, positively charged blastoff particles (α particles) that were produced by the radioactive decay of radium; α particles consist of 2 protons and two neutrons (you volition acquire more most radioactive decay in the chapter on nuclear chemistry). Rutherford and his colleagues Hans Geiger (later famous for the Geiger counter) and Ernest Marsden aimed a beam of α particles, the source of which was embedded in a atomic number 82 block to absorb most of the radiation, at a very sparse piece of aureate foil and examined the resultant handful of the α particles using a luminescent screen that glowed briefly where hitting by an α particle.

What did they discover? Most particles passed right through the foil without being deflected at all. However, some were diverted slightly, and a very small number were deflected almost straight back toward the source (Figure \(\PageIndex{iv}\)). Rutherford described finding these results: "It was quite the most incredible event that has ever happened to me in my life. It was near as incredible every bit if you fired a 15-inch vanquish at a slice of tissue paper and it came dorsum and hitting you"¹ (p. 68).

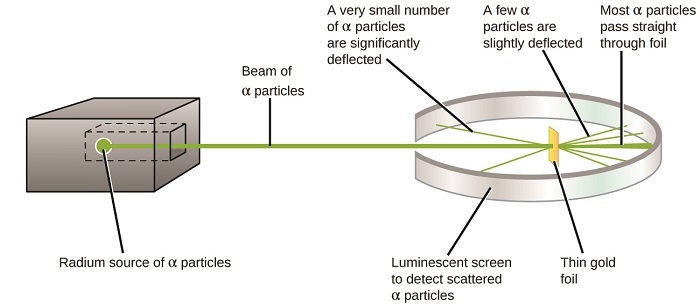
Figure \(\PageIndex{8}\): Geiger and Rutherford fired α particles at a piece of gold foil and detected where those particles went, equally shown in this schematic diagram of their experiment. Most of the particles passed directly through the foil, but a few were deflected slightly and a very small number were significantly deflected.

Here is what Rutherford deduced: Because nigh of the fast-moving α particles passed through the gold atoms undeflected, they must have traveled through essentially empty space within the atom. Alpha particles are positively charged, so deflections arose when they encountered another positive accuse (like charges repel each other). Since similar charges repel ane another, the few positively charged α particles that inverse paths abruptly must take striking, or closely approached, another body that also had a highly concentrated, positive charge. Since the deflections occurred a small fraction of the time, this accuse only occupied a modest amount of the space in the gold foil. Analyzing a serial of such experiments in detail, Rutherford drew two conclusions:

1. The book occupied by an atom must consist of a big amount of empty space.
2. A pocket-size, relatively heavy, positively charged torso, the nucleus, must be at the center of each atom.

This analysis led Rutherford to advise a model in which an atom consists of a very small, positively charged nucleus, in which nigh of the mass of the cantlet is full-bodied, surrounded by the negatively charged electrons, then that the cantlet is electrically neutral (Figure \(\PageIndex{5}\)).

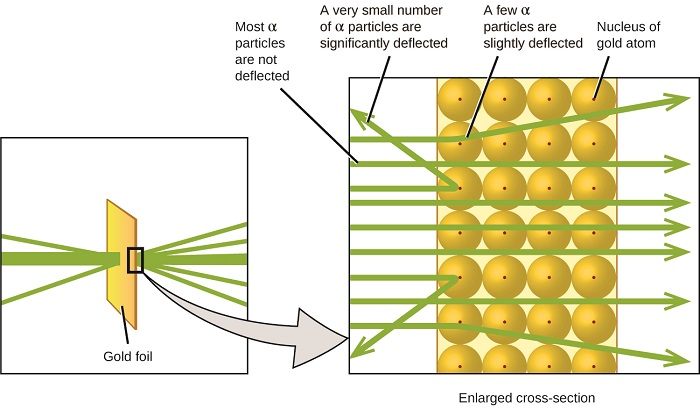
Figure \(\PageIndex{9}\): The α particles are deflected merely when they collide with or pass shut to the much heavier, positively charged gold nucleus. Considering the nucleus is very small compared to the size of an atom, very few α particles are deflected. Most pass through the relatively large region occupied past electrons, which are as well light to deflect the rapidly moving particles.

After many more experiments, Rutherford as well discovered that the nuclei of other elements contain the hydrogen nucleus as a "edifice block," and he named this more fundamental particle the proton, the positively charged, subatomic particle constitute in the nucleus. With 1 addition, which you will learn next, this nuclear model of the cantlet, proposed over a century ago, is still used today.

Some other of import finding was the discovery of isotopes. During the early 1900s, scientists identified several substances that appeared to be new elements, isolating them from radioactive ores. For example, a "new chemical element" produced by the radioactive decay of thorium was initially given the name mesothorium. However, a more detailed analysis showed that mesothorium was chemically identical to radium (another decay product), despite having a different atomic mass. This result, along with similar findings for other elements, led the English chemist Frederick Soddy to realize that an element could accept types of atoms with different masses that were chemically indistinguishable. These different types are called isotopes—atoms of the same chemical element that differ in mass. Soddy was awarded the Nobel Prize in Chemical science in 1921 for this discovery.

I puzzle remained: The nucleus was known to incorporate almost all of the mass of an atom, with the number of protons only providing half, or less, of that mass. Different proposals were made to explicate what constituted the remaining mass, including the beingness of neutral particles in the nucleus. As you lot might expect, detecting uncharged particles is very challenging, and information technology was not until 1932 that James Chadwick found evidence of neutrons, uncharged, subatomic particles with a mass approximately the same equally that of protons. The existence of the neutron also explained isotopes: They differ in mass because they have different numbers of neutrons, but they are chemically identical considering they have the same number of protons. This will exist explained in more particular later in this unit.

Video \(\PageIndex{2}\): An Introduction to Subatomic Particles

Summary

Video \(\PageIndex{3}\): A summary of discoveries in atomic theory.

Video \(\PageIndex{4}\): A different summary of discoveries in diminutive theory.

The ancient Greeks proposed that affair consists of extremely small particles called atoms. Dalton postulated that each element has a characteristic type of atom that differs in backdrop from atoms of all other elements, and that atoms of unlike elements can combine in fixed, pocket-size, whole-number ratios to class compounds. Samples of a particular compound all have the aforementioned elemental proportions by mass. When ii elements form different compounds, a given mass of one element will combine with masses of the other element in a small, whole-number ratio. During whatsoever chemical alter, atoms are neither created nor destroyed.

Although no one has actually seen the inside of an cantlet, experiments have demonstrated much about atomic structure. Thomson's cathode ray tube showed that atoms contain small, negatively charged particles called electrons. Millikan discovered that there is a fundamental electric accuse—the charge of an electron. Rutherford's gold foil experiment showed that atoms take a small, dense, positively charged nucleus; the positively charged particles inside the nucleus are called protons. Chadwick discovered that the nucleus also contains neutral particles called neutrons. Soddy demonstrated that atoms of the same element can differ in mass; these are chosen isotopes.

Footnotes

1. Ernest Rutherford, "The Development of the Theory of Atomic Structure," ed. J. A. Ratcliffe, in Background to Modern Science, eds. Joseph Needham and Walter Pagel, (Cambridge, UK: Cambridge Academy Press, 1938), 61–74. Accessed September 22, 2014, https://ia600508.us.archive.org/3/it...e032734mbp.pdf.

Glossary

Dalton's diminutive theory

set of postulates that established the fundamental properties of atoms

law of constant limerick

(also, police of definite proportions) all samples of a pure compound contain the aforementioned elements in the same proportions by mass

law of multiple proportions

when two elements react to form more than one compound, a fixed mass of ane element will react with masses of the other element in a ratio of small whole numbers

police force of definite proportions

(too, law of constant composition) all samples of a pure chemical compound contain the same elements in the same proportions by mass

blastoff particle (α particle)

positively charged particle consisting of two protons and 2 neutrons

electron

negatively charged, subatomic particle of relatively depression mass located outside the nucleus

isotopes

atoms that incorporate the same number of protons but different numbers of neutrons

neutron

uncharged, subatomic particle located in the nucleus

proton

positively charged, subatomic particle located in the nucleus

nucleus

massive, positively charged eye of an atom made up of protons and neutrons

Contributors

• Paul Flowers (University of North Carolina - Pembroke), Klaus Theopold (University of Delaware) and Richard Langley (Stephen F. Austin State University) with contributing authors.Textbook content produced by OpenStax Higher is licensed nether a Creative Commons Attribution License 4.0 license. Download for complimentary at http://cnx.org/contents/85abf193-2bd...a7ac8df6@9.110).

• Adelaide Clark, Oregon Institute of Technology
• Crash Grade Chemistry: Crash Course is a division of Complexly and videos are free to stream for educational purposes.
• TED-Ed'south commitment to creating lessons worth sharing is an extension of TED's mission of spreading swell ideas. Within TED-Ed's growing library of TED-Ed animations, you will discover carefully curated educational videos, many of which represent collaborations between talented educators and animators nominated through the TED-Ed website.

Feedback

Have feedback to give about this text? Click here.

Found a typo and want actress credit? Click here.

joubertessfull.blogspot.com

Source: https://chem.libretexts.org/Courses/Oregon_Institute_of_Technology/OIT:_CHE_201_-_General_Chemistry_I_%28Anthony_and_Clark%29/Unit_2:_The_Structure_of_the_Atom/2.1:_A_History_of_Atomic_Theory

Share this post